This is possible when y is greater than y ≥ 5 Hence, the range of f(x) is 5, ∞) Example 2 Find the domain and range of a function f(x) = (2x – 1)/(x 4) Solution Given function is f(x) = (2x – 1)/(x 4) We know that the domain of a function is the set of input values for f, in which the function is real and definedWrite the domain and range in setbuilder notation and interval notation find the inverse of f(x)=(1/2x2)sq Determine whether it is function and state its domain and range asked in ALGEBRA 2 by payton Apprentice domainofafunction
2
Y=x^2-2x-3 domain and range
Y=x^2-2x-3 domain and range- Find the domain of each of the following functions given by f(x) = (x^3 x 3)/(x^2 1) asked in Sets, Relations and Functions by RamanKumar ( 500k points) setsAlgebra Find the Domain and Range f (x)=x^22x3 f (x) = x2 − 2x − 3 f ( x) = x 2 2 x 3 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation
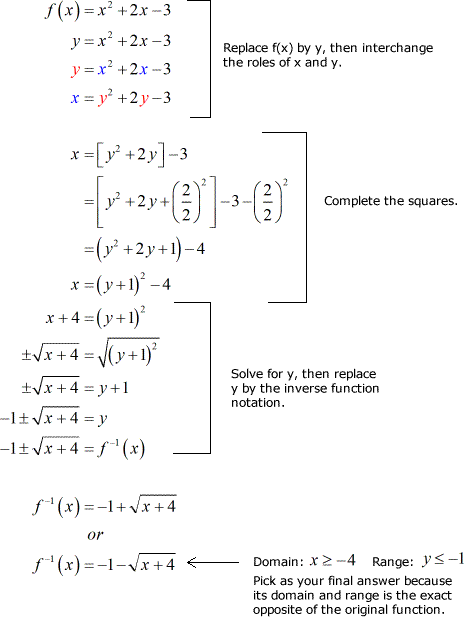



Inverse Of Quadratic Function Chilimath
Stack Exchange network consists of 178 Q&A communities including Stack Overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers Visit Stack ExchangeQuestion Given the function, f (x)= 2/x^22x3 find tthe domain and range by showing your work After that, find the x and y intercepts Answer by Fombitz () ( Show Source ) You can put this solution on YOUR website!300 seconds Q The graph of an exponential function is shown on the grid Based on the graph, which statement about the function is true?
That is, you need 1 x − y 2 ≥ 0 or "in more simple terms" 1 x ≥ y 2 So ( f ( x, y)) = { 1 x ≥ y 2 ∣ x, y ∈ R } The range is slightly more tricky but not so much Since x and y are allowed to be anything in R (so long as they are in the domain), we can work out a way for all of nonnegative R to be mapped toThe domain is where x 2 − 2 x − 8 is real, so the domain is x ≥ 4 or x ≤ 2 As for the range, if the square root function returns only the positive root, the range is y ≥ 0 To see this, note that 0 is in the range ( x = − 2 , x = 4 ) , that the function is continuous over the domain of x (so every y between the minimum and maximum is in the range), and the lim x → ∞ x 2 − 2 x − 8 = lim x → −Now y = 2x^2 2domain of x is all real numbers as abovebut as far as range is concerned we see that since square of x is always positive,its minimum value can be 0hence (square of x) has a maximum value of 0hence has a maximum value of 1 hence y has a maximum value of 2 hence range of y is all real numbers less than or equal to 2
So, its domain of f(x) is all real numbers But value of f(x) can not be less than 1 because for x = 0 the value of f(x) is 1 and also for any values of x, the value of f(x) can never be less than 1 So, Range of f(x) is all real numbers greater than 0 Hence, Domain and Range of f(x) is given by Domain {x all real numbers} ;The same applies to the vertical extent of the graph, so the domain and range include all real numbers Figure 18 For the reciprocal function f ( x) = 1 x, f ( x) = 1 x, we cannot divide by 0, so we must exclude 0 from the domain Further, 1 divided by any value can never be 0, so the range also will not include 0Which integer is not in the range?



Find The Domain And Range Of Each Of The Following Gauthmath



Domain And Range Examples Domain And Range Of A Function
Domain and range of z = x^2 y^2 WolframAlpha Volume of a cylinder?Question 3 Find the domain and range of y = 2 arcsin(3 x 1) Solution to question 3 1 Domain To find the domain, we need to impose the following condition 1 ≤ (3 x 1) ≤ 1 solve to obtain domain as 0 ≤ x ≤ 2 / 3 2 Range The range of arcsin(3x 1) is the same as the range of arcsin(x) which is pi / 2 ≤ y ≤ pi / 2 HenceAlgebra Find the Domain and Range y=x^23 y = x2 3 y = x 2 3 The domain of the expression is all real numbers except where the expression is undefined In this case, there is no real number that makes the expression undefined Interval Notation (−∞,∞) ( ∞, ∞) Set Builder Notation {xx ∈ R} { x x ∈ ℝ }




Domain And Range Of 1 X 2 2x 8 Youtube
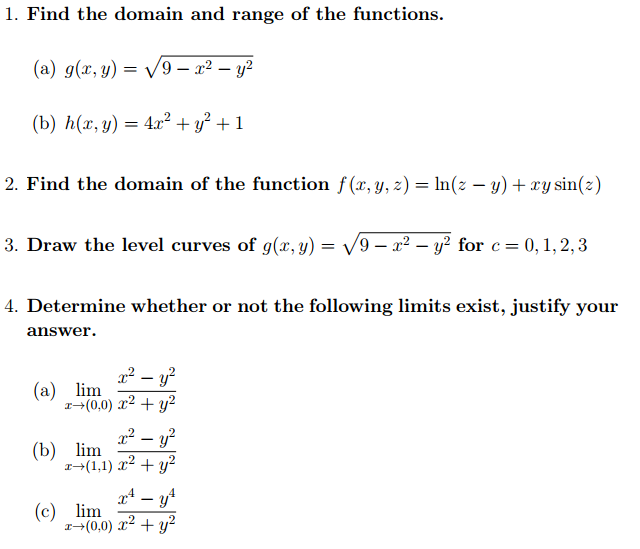



Solved Find The Domain And Range Of The Functions G X Y Chegg Com
3 = 2 Thus, we can write our range in the following two ways R (1 ;2) (2;1) (10) R fyjy 6= 2 g (11) Notice The function is graphed in red and the vertical line in blue is our asymptote where our domain gap is as well Example 2 h(x) = p x 4 (12) We don't have any fractions or logs here but we do have a square root and we knowAnswer choices The range is the set of all real numbers less than 0 The domain is the set of all real numbers greater than 4 The range is the set of all real numbers greater than 0Find stepbystep Algebra 2 solutions and your answer to the following textbook question What are the domain and range of each function?



2



1
So the Domain is all Real numbers except x = 3 Range all the values that y will be after we put all the domain values ( some time it could be a bit tricky ) One way to find the Range is to Solve for x ( find the inverse ) y(x3) = 2 → yx 3y = 2 → yx = 23y → x = (23y)/y So, y can not be 0 that means the Range is all real numbers Except y = 0 Brainly User for domain x2 is not equal to zero so x is not equal to 2 domain is R {2} its range is {1,1} because it will give only 1 or 1 for any x belongs to R {2} we have function x2/lx2l we know that lx2l= (x2) according to values of x so (x2)/ (x2) =1A Relation R is given by the set {(x, y)/y = x 3, x ∈ {0, 1, 2, 3, 4, 5}} Determine its domain and range
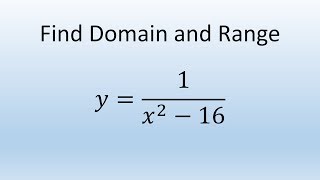



Find Domain And Range Of Function Y 1 X 2 16 Youtube




6 Ways To Find The Domain Of A Function Wikihow
Cara Mencari Domain dan Range Fungsi Setiap fungsi memiliki dua variabel, yaitu variabel bebas dan variabel terikat Secara harfiah nilai variabel terikat "tergantung" pada variabel bebas Sebagai contoh, dalam fungsi y = f(x) = 2x y, xThe parabola has a maximum value at y = 2 and it can go down as low as it wants The range is simply y ≤ 2 The summary of domain and range is the following Example 4 Find the domain and range of the quadratic function y = {x^2} 4x 1 Just like our previous examples, a quadratic function will always have a domain of all x valuesThe function never goes below 0 So f of x so 0 is less than or equal to f of x It does equal 0 right over here f of negative 4 is 0 And then the highest y value or the highest value that f of x obtains in this function definition is 8 f of 7 is 8 It never gets above 8, but it does equal 8 right over here when x is equal to 7
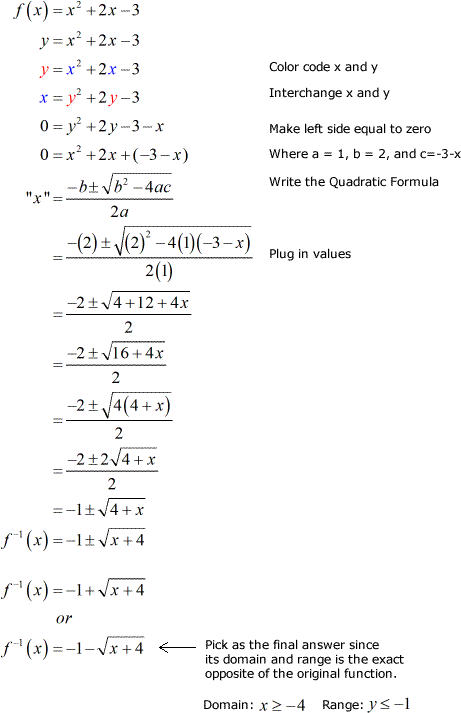



Inverse Of Quadratic Function Chilimath




What Is The Range And The Domain Of F X X2 2x 3 Brainly Com


