Form the table, it is observed that, the rate of change between x and y is 3 This can be written using the linear function y= x3 Linear Function Formula The expression for the linear function is the formula to graph a straight line The expression for the linear equation is;A linear equation is represented as a line graph In order to draw the line graph we require several pairs of coordinates These coordinates represent the relationship given in the equationX – 4y 4 y x 1 On a coordinate plane, 2 straight lines are shown The first solid line has a positive slope and goes through (0, negative 1) and (4, 0) Everything above the line is shaded
Graphing Linear Functions Geogebra
Linear graph y=x+3
Linear graph y=x+3-Advertisement For this example, it's simplest to first solve for "y =" This is especially true if I'm using a graphing calculator to fill in my Tchart, because graphing calculators can only handle line equations when they're in the form "y =" So, to make my like easier, I'll solve thisWhen x is 0, y is already 1 So 1 is also needed;
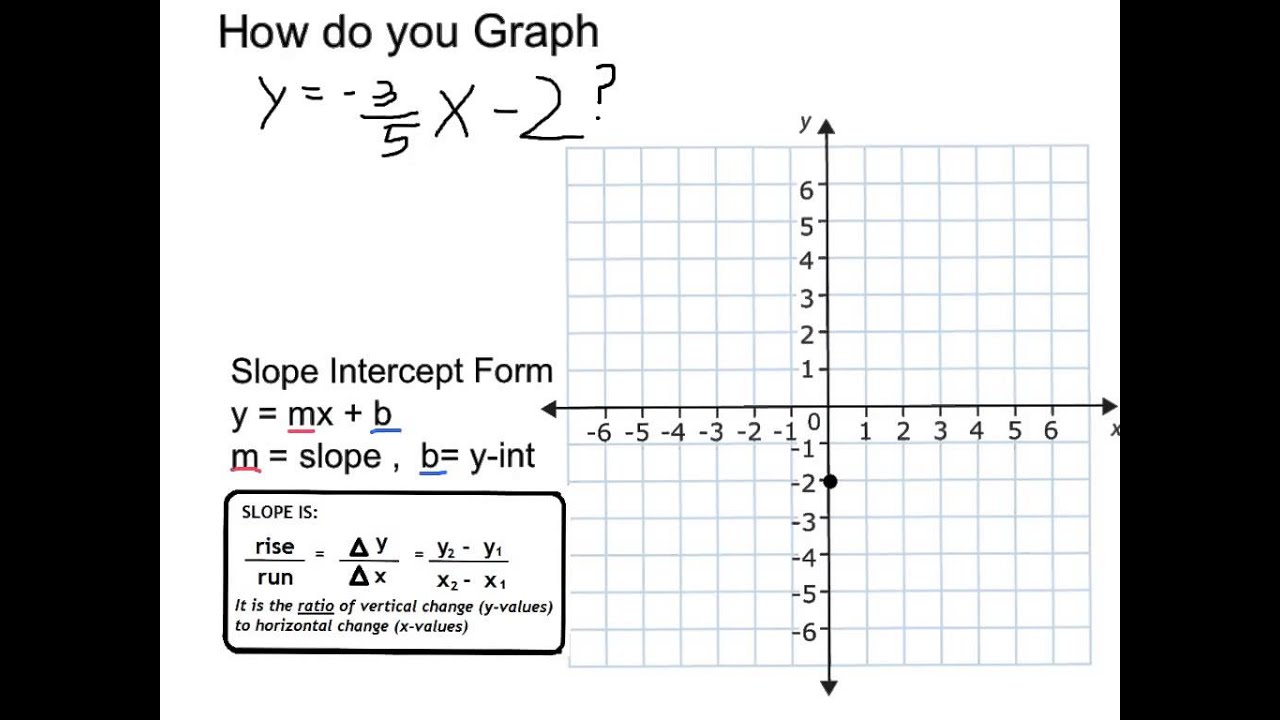



Graph Y 3 5 X 2 Youtube
Method 1 using two points to graph a linear equation The graph of any linear equation like y = 3 x 2 or y = − x 9 is a line, and only two points are needed to define a line The idea with this method is to find two points on the line by picking values of xAnd so y = 2x 1;Use the intercept method to graph xy=3 Step 1 Intercepts occur when one of the coordinates is equal to 0 So the yintercept occurs at x=0 and the xintercept occurs at y=0 Step 2 It takes two points to make a line So use these intercepts points Step 3 When x=0, then 0y=3 or y=3 So we have point (0,3) Step 4 When y=0, then x0=3 or
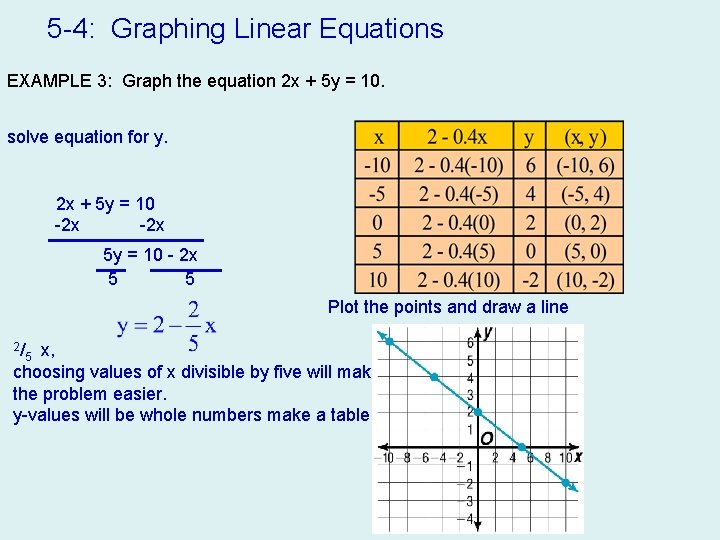
In example 3 look at the tables of values and note that for a given value of x, the value of y in the equation y = 3x 2 is two more than the corresponding value of y in the equation y = 3x Look now at the graphs of the two equations and note that the graph of y = 3x 2 seems to have the same slope as yThen click on the graph until the correct one is displayed 3 x y 1 = 0 / < It goes from the bottom left corner up into the top right corner Using graph paper, graph the following equation Then click on the graph until the correct one is displayed y = 3/5 x 12 (a) Complete the table of values for y = 1 – 2x (Total for question 2 is 5 marks) (b) On the grid draw the graph of y = 1 – 2 x for values of x from –2 to 3
Here are some example valuesY = mx c where m is the slope, c is the intercept and (x,y) areSolution for Graph each linear equation 3 x3y= 6 Q A merchant sold his entire stock of shirts and ties for $1000, the shirts being priced at 3 for $10 A Let the number of shirt sold x units Let the number of ties sold y unit
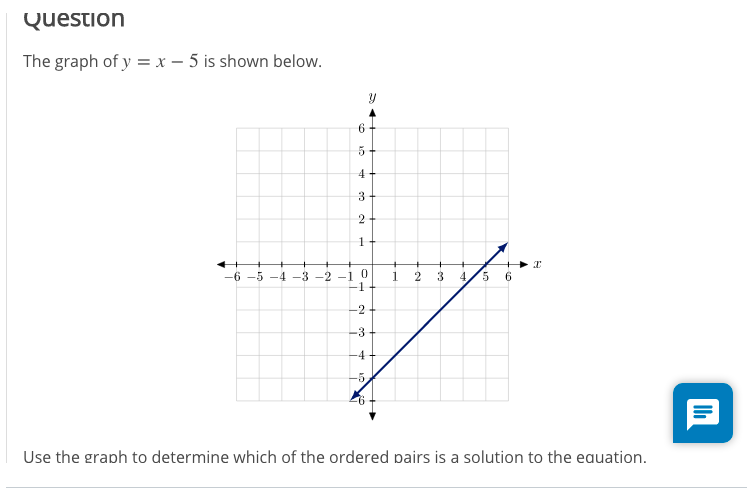



Question The Graph Of Y X 5 Is Shown Below Y 6 4 Chegg Com




A System Of Linear Equations See How To Solve It At Qanda
2 Find the value of p that makes the linear graph y = p − 3x pass through the point where the lines 4x − y =6 and 2x − 5y = 12 intersect 3 Faced with the problem of multiplying 5 6 times 5 3, Brook is having trouble deciding which of these four answers is correct 5 18,5 9,25 18,or 25 9 Your help is needed Once you have answered Brook's question, experiment with other examples ofFree graphing calculator instantly graphs your math problemsWe designate (3, 5) as (x 2, y 2) and (4, 2) as (x 1, y 1) Substituting into Equation (1) yields Note that we get the same result if we subsitute 4 and 2 for x 2 and y 2 and 3 and 5 for x 1 and y 1 Lines with various slopes are shown in Figure 78 below



Graph The Linear Equation Yx 2 1 Draw



Quadratics Graphing Parabolas Sparknotes
Steps for Solving Linear Equation y = 5x3 y = − 5 x 3 Swap sides so that all variable terms are on the left hand side Swap sides so that all variable terms are on the left hand side 5x3=y − 5 x 3 = y Subtract 3 from both sides Subtract 3 from both sides− q a) The x intercept is obtained by letting y = 0Linear equations A linear equation is an equation that represents a straight line When plotted on a graph, a linear equation always results in a straight line, as in the figure below, which is a special case of a linear equation referred to as the identity function, y = x




Graphing Systems Of Linear Inequalities



Quadratics Graphing Parabolas Sparknotes
Question graph the line y=x3 Answer by jim_thompson5910 () ( Show Source ) You can put this solution on YOUR website!As long as you do your work neatly and orderly, you shouldn't have much trouble with graphing linear equations Here are some more examples Graph First I'll do my Tchart Since I am multiplying the variable x by a fraction that has 3 as its denominator, I will pick xvalues that are multiples of 3 This wayClick here👆to get an answer to your question ️ Draw the graph for the linear equation given below 2x 3y = 4 Join / Login > 9th > Maths > Linear Equations in Two Variable > Graph of a Linear Equation in Two Variables Draw the graph for the each linear equation given below y = 2 3 x
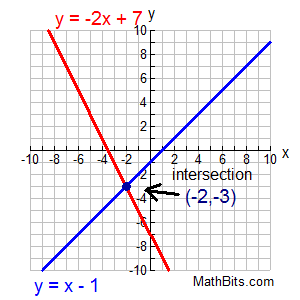



Systems Of Linear Equations Graphical Solution Mathbitsnotebook A1 Ccss Math
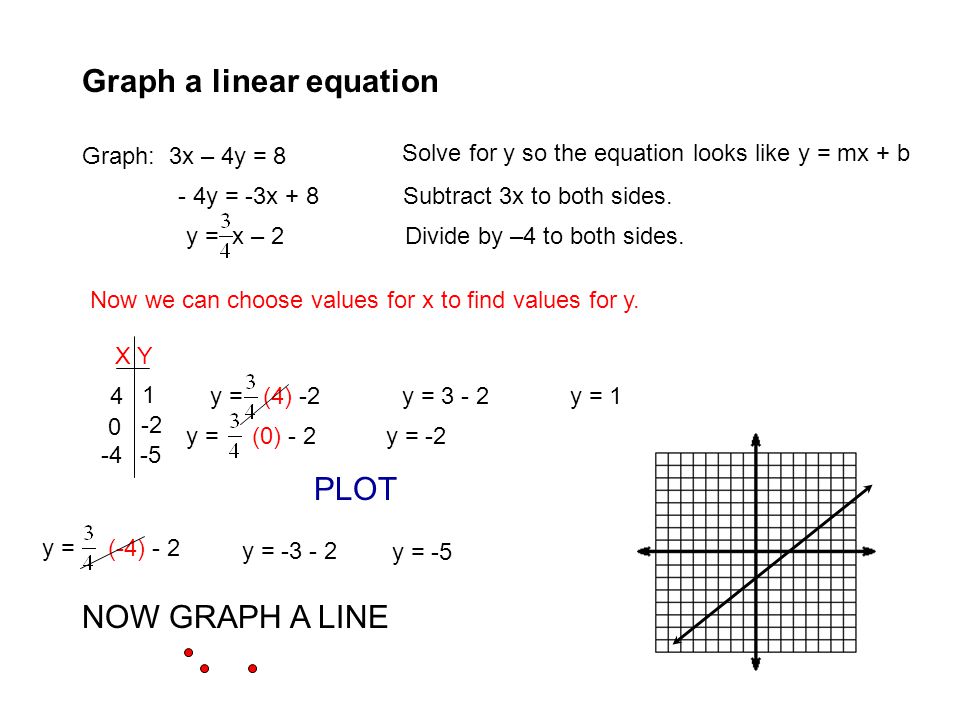



Graph A Linear Equation Graph 2x 3y 12 Solve For Y So The Equation Looks Like Y Mx B 3y 2x 12 Subtract 2x To Both Sides Y X 4 Divide Ppt Download
For example, Use your graph to solve the equation x 2 x – 2 = 0 We have already drawn the graph for y = x 2 x – 2 The other side of our equation is zero, so we need to think about the line y = 0 This is the xaxis, so look for the points where the graph crosses the xaxis The solutions are x = 1 and x = 2 To solve the equation x 2 x – 2 = 3, we would draw the line y = 3 The graph of y = x − 3 is almost the same as y = x The difference is that every point on y = x has been lowered by 3 Thus, instead of the yintercept being at y = 0 it is at y = − 3 instead Consider the generic equation of y = mx c where m is the gradient (slope) If you compare this to both y = x and y = x − 3 you will observe that there is no value shown for m (1 point) x = 2, 0, 2, 4 y = 4, 0, 4, 8 The values do not show a linear function Yes, they show a linear Math The graph represents function 1 and the equation represents function 2 A graph with numbers 0 to 4 on the xaxis and yaxis at increments of 1 A horizontal straight line is drawn joining the ordered pairs 0, 3 and 4, 3
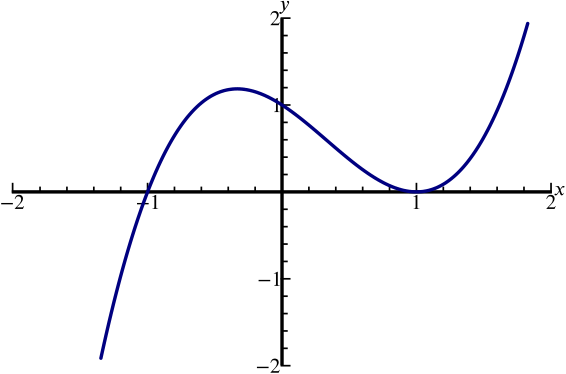



Can We Sketch The Graph Y X 3 X 2 X 1 Polynomials Rational Functions Underground Mathematics
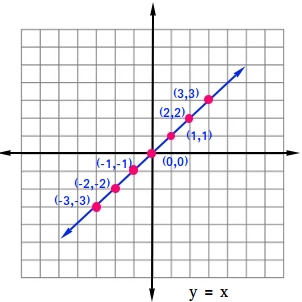



Linear Parent Function


